An 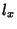 table is a tabulation of numbers which is used to calculate life expectancies.
table is a tabulation of numbers which is used to calculate life expectancies.
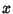 |
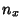 |
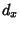 |
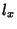 |
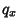 |
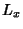 |
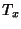 |
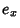 |
| 0 |
1000 |
200 |
1.00 |
0.20 |
0.90 |
2.70 |
2.70 |
| 1 |
800 |
100 |
0.80 |
0.12 |
0.75 |
1.80 |
2.25 |
| 2 |
700 |
200 |
0.70 |
0.29 |
0.60 |
1.05 |
1.50 |
| 3 |
500 |
300 |
0.50 |
0.60 |
0.35 |
0.45 |
0.90 |
| 4 |
200 |
200 |
0.20 |
1.00 |
0.10 |
0.10 |
0.50 |
| 5 |
0 |
0 |
0.00 |
-- |
0.00 |
0.00 |
-- |
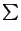 |
|
1000 |
2.70 |
|
|
|
|
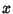 : Age category (
: Age category (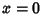 , 1, ...,
, 1, ..., 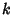 ). These values can be in any convenient units, but must be chosen
so that no observed lifespan extends past category
). These values can be in any convenient units, but must be chosen
so that no observed lifespan extends past category 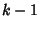 .
.
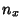 : Census size, defined as the number of individuals in the study population who survive to the
beginning of age category
: Census size, defined as the number of individuals in the study population who survive to the
beginning of age category 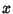 . Therefore,
. Therefore, 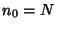 (the total population size) and
(the total population size) and 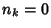 .
.
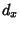 :
: 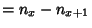 ;
;
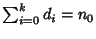 . Crude death rate, which measures the number of individuals
who die within age category
. Crude death rate, which measures the number of individuals
who die within age category 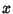 .
.
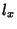 :
: 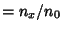 . Survivorship, which measures the proportion of individuals who survive to the
beginning of age category
. Survivorship, which measures the proportion of individuals who survive to the
beginning of age category 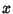 .
.
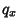 :
: 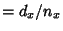 ;
; 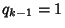 . Proportional death rate, or ``risk,'' which measures the proportion of
individuals surviving to the beginning of age category
. Proportional death rate, or ``risk,'' which measures the proportion of
individuals surviving to the beginning of age category 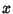 who die within that category.
who die within that category.
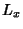 :
:
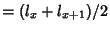 . Midpoint survivorship, which measures the proportion of individuals
surviving to the midpoint of age category
. Midpoint survivorship, which measures the proportion of individuals
surviving to the midpoint of age category 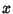 . Note that the simple averaging formula must be replaced
by a more complicated expression if survivorship is nonlinear within age categories. The
sum
. Note that the simple averaging formula must be replaced
by a more complicated expression if survivorship is nonlinear within age categories. The
sum
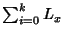 gives the total number of age categories lived by the entire study population.
gives the total number of age categories lived by the entire study population.
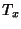 :
:
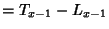 ;
;
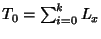 . Measures the total number of age categories left to be
lived by all individuals who survive to the beginning of age category
. Measures the total number of age categories left to be
lived by all individuals who survive to the beginning of age category 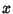 .
.
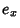 :
: 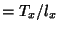 ;
; 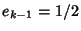 . Life expectancy, which is the mean number of age categories remaining until
death for individuals surviving to the beginning of age category
. Life expectancy, which is the mean number of age categories remaining until
death for individuals surviving to the beginning of age category 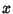 .
.
For all 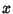 ,
, 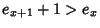 . This means that the total expected lifespan increases monotonically. For instance,
in the table above, the one-year-olds have an average age at death of
. This means that the total expected lifespan increases monotonically. For instance,
in the table above, the one-year-olds have an average age at death of 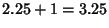 , compared to 2.70 for newborns.
In effect, the age of death of older individuals is a distribution conditioned on the fact that they have survived
to their present age.
, compared to 2.70 for newborns.
In effect, the age of death of older individuals is a distribution conditioned on the fact that they have survived
to their present age.
It is common to study survivorship as a semilog plot of 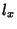 vs.
vs. 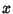 , known as a Survivorship Curve.
A so-called
, known as a Survivorship Curve.
A so-called 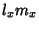 table can be used to calculate the mean generation time of a population. Two
table can be used to calculate the mean generation time of a population. Two 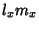 tables are illustrated below.
tables are illustrated below.
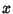 |
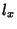 |
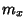 |
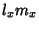 |
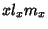 |
| 0 |
1.00 |
0.00 |
0.00 |
0.00 |
| 1 |
0.70 |
0.50 |
0.35 |
0.35 |
| 2 |
0.50 |
1.50 |
0.75 |
1.50 |
| 3 |
0.20 |
0.00 |
0.00 |
0.00 |
| 4 |
0.00 |
0.00 |
0.00 |
0.00 |
| |
|
|
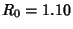 |
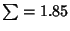 |
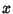 |
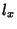 |
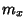 |
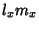 |
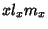 |
| 0 |
1.00 |
0.00 |
0.00 |
0.00 |
| 1 |
0.70 |
0.00 |
0.00 |
0.00 |
| 2 |
0.50 |
2.00 |
1.00 |
2.00 |
| 3 |
0.20 |
0.50 |
0.10 |
0.30 |
| 4 |
0.00 |
0.00 |
0.00 |
0.00 |
| |
|
|
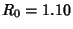 |
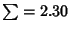 |
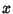 : Age category (
: Age category (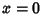 , 1, ...,
, 1, ..., 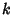 ). These values can be in any convenient units, but must be chosen
so that no observed lifespan extends past category
). These values can be in any convenient units, but must be chosen
so that no observed lifespan extends past category 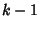 (as in an
(as in an 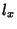 table).
table).
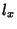 :
: 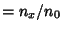 . Survivorship, which measures the proportion of individuals who survive to the
beginning of age category
. Survivorship, which measures the proportion of individuals who survive to the
beginning of age category 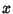 (as in an
(as in an 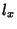 table).
table).
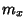 : The average number of offspring produced by an individual in age category
: The average number of offspring produced by an individual in age category 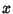 while in that age
category.
while in that age
category.
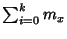 therefore represents the average lifetime number of offspring produced by an
individual of maximum lifespan.
therefore represents the average lifetime number of offspring produced by an
individual of maximum lifespan.
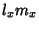 : The average number of offspring produced by an individual within age category
: The average number of offspring produced by an individual within age category 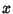 weighted by the probability of surviving to the beginning of that age category.
weighted by the probability of surviving to the beginning of that age category.
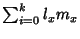 therefore
represents the average lifetime number of offspring produced by a member of the study population. It is called
the net reproductive rate per generation and is often denoted
therefore
represents the average lifetime number of offspring produced by a member of the study population. It is called
the net reproductive rate per generation and is often denoted 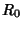 .
.
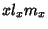 : A column weighting the offspring counted in the previous column by their parents' age when they
were born. Therefore, the ratio
: A column weighting the offspring counted in the previous column by their parents' age when they
were born. Therefore, the ratio
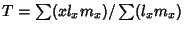 is the mean generation time of the population.
is the mean generation time of the population.
The Malthusian Parameter 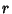 measures the reproductive rate per unit time and can be calculated as
measures the reproductive rate per unit time and can be calculated as
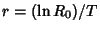 . For an exponentially increasing population, the population size
. For an exponentially increasing population, the population size 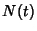 at time
at time 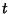 is then given
by
is then given
by
In the above two tables, the populations have identical reproductive rates of 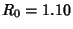 . However, the shift toward
later reproduction in population 2 increases the generation time, thus slowing the rate of Population Growth.
Often, a slight delay of reproduction decreases Population Growth more strongly than does even a fairly
large reduction in reproductive rate.
. However, the shift toward
later reproduction in population 2 increases the generation time, thus slowing the rate of Population Growth.
Often, a slight delay of reproduction decreases Population Growth more strongly than does even a fairly
large reduction in reproductive rate.
See also Gompertz Curve, Logistic Growth Curve, Makeham Curve,
Malthusian Parameter, Population Growth, Survivorship Curve
© 1996-9 Eric W. Weisstein
1999-05-25
![]() table is a tabulation of numbers which is used to calculate life expectancies.
table is a tabulation of numbers which is used to calculate life expectancies.
![]() ,
, ![]() . This means that the total expected lifespan increases monotonically. For instance,
in the table above, the one-year-olds have an average age at death of
. This means that the total expected lifespan increases monotonically. For instance,
in the table above, the one-year-olds have an average age at death of ![]() , compared to 2.70 for newborns.
In effect, the age of death of older individuals is a distribution conditioned on the fact that they have survived
to their present age.
, compared to 2.70 for newborns.
In effect, the age of death of older individuals is a distribution conditioned on the fact that they have survived
to their present age.
![]() vs.
vs. ![]() , known as a Survivorship Curve.
A so-called
, known as a Survivorship Curve.
A so-called ![]() table can be used to calculate the mean generation time of a population. Two
table can be used to calculate the mean generation time of a population. Two ![]() tables are illustrated below.
tables are illustrated below.
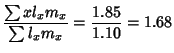
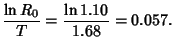
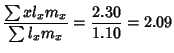
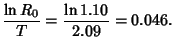
![]() measures the reproductive rate per unit time and can be calculated as
measures the reproductive rate per unit time and can be calculated as
![]() . For an exponentially increasing population, the population size
. For an exponentially increasing population, the population size ![]() at time
at time ![]() is then given
by
is then given
by