|
|
|
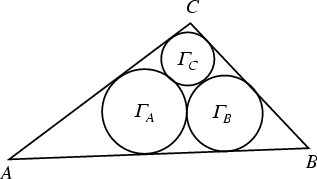
Draw within a given Triangle three Circles, each of which is Tangent to the
other two and to two sides of the Triangle. Denote the three
Circles so constructed ![]() ,
, ![]() , and
, and
![]() . Then
. Then ![]() is tangent to
is tangent to ![]() and
and ![]() ,
, ![]() is tangent to
is tangent to ![]() and
and ![]() , and
, and ![]() is tangent
to
is tangent
to ![]() and
and ![]() .
.
See also Ajima-Malfatti Points, Malfatti's Right Triangle Problem
References
Dörrie, H. ``Malfatti's Problem.'' §30 in 100 Great Problems of Elementary Mathematics: Their History and Solutions.
New York: Dover, pp. 147-151, 1965.
Forder, H. G. Higher Course Geometry. Cambridge, England: Cambridge University Press, pp. 244-245, 1931.
Fukagawa, H. and Pedoe, D. Japanese Temple Geometry Problems (San Gaku). Winnipeg: The Charles Babbage
Research Centre, pp. 106-120, 1989.
Gardner, M. Fractal Music, Hypercards, and More Mathematical Recreations from Scientific
American Magazine. New York: W. H. Freeman, pp. 163-165, 1992.
Goldberg, M. ``On the Original Malfatti Problem.'' Math. Mag. 40, 241-247, 1967.
Lob, H. and Richmond, H. W. ``On the Solution of Malfatti's Problem for a Triangle.'' Proc. London Math. Soc. 2, 287-304, 1930.
Woods, F. S. Higher Geometry. New York: Dover, pp. 206-209, 1961.