|
|
|
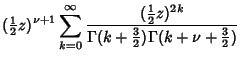 |
|||
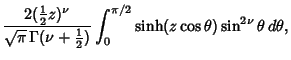 |
See also Anger Function, Struve Function, Weber Functions
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Modified Struve Function
![]() .'' §12.2 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 498, 1972.
.'' §12.2 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 498, 1972.