|
|
|
The modulus of a Complex Number ![]() is denoted
is denoted ![]() .
.
| (1) |
| (2) |
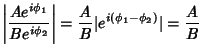 |
(3) | ||
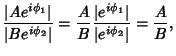 |
(4) |
| (5) |
| (6) | |||
| (7) |
| (8) |
| (9) |
The only functions satisfying identities of the form
| (10) |
See also Absolute Square
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 16, 1972.
Robinson, R. M. ``A Curious Mathematical Identity.'' Amer. Math. Monthly 64, 83-85, 1957.