|
|
|
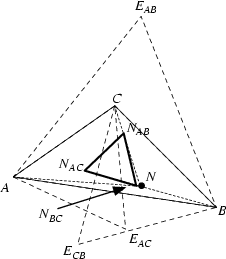
The inner Napoleon point ![]() is the Concurrence of lines drawn between Vertices of a given Triangle
is the Concurrence of lines drawn between Vertices of a given Triangle ![]() and the opposite Vertices of the corresponding
inner Napoleon Triangle
and the opposite Vertices of the corresponding
inner Napoleon Triangle
![]() . The Triangle Center Function of the
inner Napoleon point is
. The Triangle Center Function of the
inner Napoleon point is
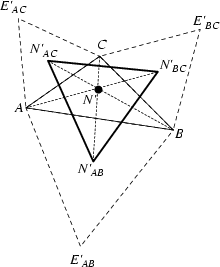
The outer Napoleon point ![]() is the Concurrence of lines drawn between Vertices of a given Triangle
is the Concurrence of lines drawn between Vertices of a given Triangle ![]() and the opposite Vertices of the corresponding
outer Napoleon Triangle
and the opposite Vertices of the corresponding
outer Napoleon Triangle
![]() . The Triangle Center Function of
the point is
. The Triangle Center Function of
the point is
See also Napoleon's Theorem, Napoleon Triangles
References
Casey, J. Analytic Geometry, 2nd ed. Dublin: Hodges, Figgis, & Co., pp. 442-444, 1893.
Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994.