|
|
|
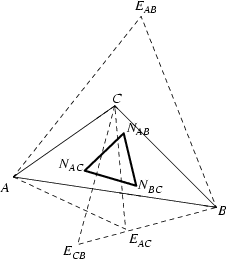
The inner Napoleon triangle is the Triangle
![]() formed by the centers of internally erected
Equilateral Triangles
formed by the centers of internally erected
Equilateral Triangles
![]() ,
,
![]() , and
, and
![]() on the sides
of a given Triangle
on the sides
of a given Triangle ![]() . It is an Equilateral Triangle.
. It is an Equilateral Triangle.
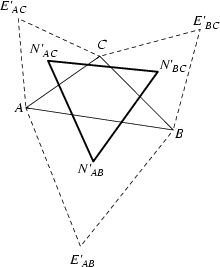
The outer Napoleon triangle is the Triangle
![]() formed by the centers of externally erected
Equilateral Triangles
formed by the centers of externally erected
Equilateral Triangles
![]() ,
,
![]() , and
, and
![]() on the
sides of a given Triangle
on the
sides of a given Triangle ![]() . It is also an Equilateral Triangle.
. It is also an Equilateral Triangle.
See also Equilateral Triangle, Napoleon Points, Napoleon's Theorem
References
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 60-65, 1967.