|
|
|
The number of nonassociative ![]() -products with
-products with ![]() elements preceding the rightmost left parameter is
elements preceding the rightmost left parameter is
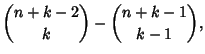 |
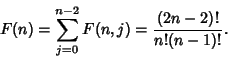
References
Niven, I. M. Mathematics of Choice: Or, How to Count Without Counting. Washington, DC: Math. Assoc. Amer.,
pp. 140-152, 1965.