An Algebra which does not satisfy
is called a nonassociative algebra. Bott and Milnor (1958) proved that the only Division
Algebras are for 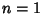 , 2, 4, and 8. Each gives rise to an Algebra with particularly useful
physical applications (which, however, is not itself necessarily nonassociative), and these four cases correspond to
Real Numbers, Complex Numbers, Quaternions, and
Cayley Numbers, respectively.
, 2, 4, and 8. Each gives rise to an Algebra with particularly useful
physical applications (which, however, is not itself necessarily nonassociative), and these four cases correspond to
Real Numbers, Complex Numbers, Quaternions, and
Cayley Numbers, respectively.
See also Algebra, Cayley Number, Complex Number, Division Algebra, Quaternion,
Real Number
References
Bott, R. and Milnor, J. ``On the Parallelizability of the Spheres.'' Bull. Amer. Math. Soc. 64, 87-89, 1958.
© 1996-9 Eric W. Weisstein
1999-05-25