|
|
|
A Function ![]() is said to be periodic with period
is said to be periodic with period ![]() if
if ![]() for
for ![]() , 2, .... For example, the
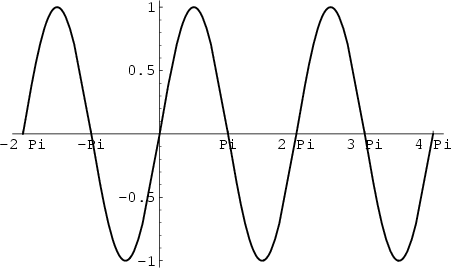
Sine function
, 2, .... For example, the
Sine function ![]() is periodic with period
is periodic with period ![]() (as well as with period
(as well as with period ![]() ,
, ![]() ,
, ![]() , etc.).
, etc.).
The Constant Function ![]() is periodic with any period
is periodic with any period ![]() for all Nonzero Real Numbers
for all Nonzero Real Numbers ![]() , so there is no concept analogous to the Least Period or a Periodic Point for constant functions.
, so there is no concept analogous to the Least Period or a Periodic Point for constant functions.
See also Periodic Point, Periodic Sequence
References
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York:
McGraw-Hill, pp. 425-427, 1953.
Spanier, J. and Oldham, K. B. ``Periodic Functions.'' Ch. 36 in An Atlas of Functions.
Washington, DC: Hemisphere, pp. 343-349, 1987.