A Congruence of the form
where 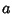 ,
, 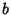 , and
, and 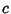 are Integers. A general quadratic congruence can be reduced to the congruence
are Integers. A general quadratic congruence can be reduced to the congruence
and can be solved using Excludents, although solution of the general polynomial congruence
is intractable.
See also Congruence, Excludent, Linear Congruence
© 1996-9 Eric W. Weisstein
1999-05-25