|
|
|
There are 17 standard-form quadratic surfaces. The general quadratic is written
| (1) |
![$\displaystyle \left[\begin{array}{ccc}a & h & g\\ h & b & f\\ g & f & c\end{array}\right]$](q_534.gif) |
(2) | ||
![$\displaystyle \left[\begin{array}{cccc}a & h & g & p\\ h & b & f & q\\ g & f & c & r\\ p & q & r & d\end{array}\right]$](q_535.gif) |
(3) | ||
| (4) | |||
| (5) | |||
| (6) |
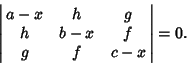 |
(7) |
| (8) |
| Surface | Equation |
|
|||
| Coincident Planes | 1 | 1 | |||
| Ellipsoid (Imaginary) |
|
3 | 4 | 1 | |
| Ellipsoid (Real) |
|
3 | 4 | 1 | |
| Elliptic Cone (Imaginary) |
|
3 | 3 | 1 | |
| Elliptic Cone (Real) |
|
3 | 3 | 0 | |
| Elliptic Cylinder (Imaginary) |
|
2 | 3 | 1 | |
| Elliptic Cylinder (Real) |
|
2 | 3 | 1 | |
| Elliptic Paraboloid |
|
2 | 4 | 1 | |
| Hyperbolic Cylinder |
|
2 | 3 | 0 | |
| Hyperbolic Paraboloid |
|
2 | 4 | 0 | |
| Hyperboloid of one Sheet |
|
3 | 4 | 0 | |
| Hyperboloid of two Sheets |
|
3 | 4 | 0 | |
| Intersecting Planes (Imaginary) |
|
2 | 2 | 1 | |
| Intersecting Planes (Real) |
|
2 | 2 | 0 | |
| Parabolic Cylinder | 1 | 3 | |||
| Parallel Planes (Imaginary) | 1 | 2 | |||
| Parallel Planes (Real) | 1 | 2 |
See also Cubic Surface, Ellipsoid, Elliptic Cone, Elliptic Cylinder, Elliptic Paraboloid, Hyperbolic Cylinder, Hyperbolic Paraboloid, Hyperboloid, Plane, Quartic Surface, Surface
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 210-211, 1987.
|
|
|
© 1996-9 Eric W. Weisstein