where
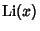 is the Logarithmic Integral, the sum is taken over all nontrivial zeros
is the Logarithmic Integral, the sum is taken over all nontrivial zeros 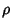 (i.e., those other than
(i.e., those other than
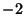 ,
, 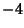 , ...) of the Riemann Zeta Function
, ...) of the Riemann Zeta Function 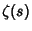 , and
, and 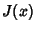 is Riemann Weighted Prime-Power Counting
Function.
is Riemann Weighted Prime-Power Counting
Function.
See also Logarithmic Integral, Prime Number Theorem, Riemann Weighted Prime-Power Counting Function,
Riemann Zeta Function
© 1996-9 Eric W. Weisstein
1999-05-25