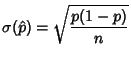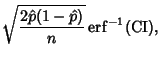Let there be 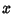 successes out of
successes out of 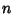 Bernoulli Trials. The sample proportion is the fraction of samples which
were successes, so
Bernoulli Trials. The sample proportion is the fraction of samples which
were successes, so
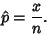 |
(1) |
For large 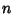 ,
, 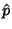 has an approximately Normal Distribution.
Let RE be the Relative Error and SE the Standard Error, then
has an approximately Normal Distribution.
Let RE be the Relative Error and SE the Standard Error, then
where CI is the Confidence Interval and
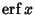 is the Erf function. The number of tries needed to determine
is the Erf function. The number of tries needed to determine 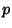 with Relative Error RE and Confidence Interval CI is
with Relative Error RE and Confidence Interval CI is
![\begin{displaymath}
n={2[\mathop{\rm erf}\nolimits ^{-1}(\mathop{\rm CI}\nolimits )]^2\hat p(1-\hat p)\over (\mathop{\rm RE})^2}.
\end{displaymath}](s1_86.gif) |
(5) |
© 1996-9 Eric W. Weisstein
1999-05-26
![]() successes out of
successes out of ![]() Bernoulli Trials. The sample proportion is the fraction of samples which
were successes, so
Bernoulli Trials. The sample proportion is the fraction of samples which
were successes, so