|
|
|
The Schwarz triangles are Spherical Triangles which, by repeated reflection in their indices, lead to a set of congruent Spherical Triangles covering the Sphere a finite number of times.
Schwarz triangles are specified by triples of numbers ![]() . There are four ``families'' of Schwarz triangles, and the
largest triangles from each of these families are
. There are four ``families'' of Schwarz triangles, and the
largest triangles from each of these families are
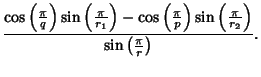 |
See also Colunar Triangle, Spherical Triangle
References
Coxeter, H. S. M. Regular Polytopes, 3rd ed. New York: Dover, pp. 112-113 and 296, 1973.
Schwarz, H. A. ``Zur Theorie der hypergeometrischen Reihe.'' J. reine angew. Math.
75, 292-335, 1873.