|
|
|
The Natural Norm induced by the L2-Norm. Let
![]() be the Adjoint of the Square Matrix
be the Adjoint of the Square Matrix
![]() , so that
, so that
![]() , then
, then
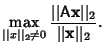 |
See also L2-Norm, Matrix Norm
References
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 5th ed.
San Diego, CA: Academic Press, pp. 1115, 1979.
Strang, G. §6.2 and 7.2 in Linear Algebra and Its Applications, 4th ed. New York: Academic Press, 1980.