|
|
|
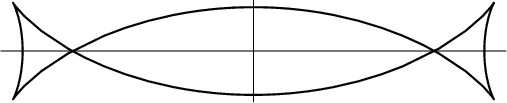
A curve investigated by Talbot which is the Negative Pedal Curve of an Ellipse with respect to its center.
It has four Cusps and two Nodes, provided the Eccentricity of
the Ellipse is greater than ![]() . Its Cartesian Equation is
. Its Cartesian Equation is
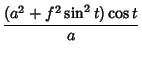 |
|||
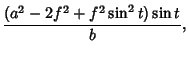 |
References
Lockwood, E. H. A Book of Curves. Cambridge, England: Cambridge University Press, p. 157, 1967.
MacTutor History of Mathematics Archive. ``Talbot's Curve.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Talbots.html.