|
|
|
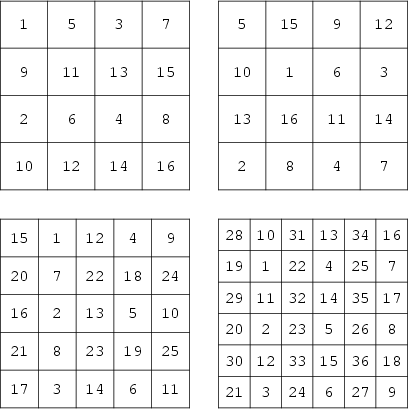
An ![]() Array of the integers from 1 to
Array of the integers from 1 to ![]() such that the difference between any one integer and its
neighbor (horizontally, vertically, or diagonally, without wrapping around) is greater than or equal to some value
such that the difference between any one integer and its
neighbor (horizontally, vertically, or diagonally, without wrapping around) is greater than or equal to some value ![]() is called
a
is called
a ![]() -talisman square. The above illustrations show (4, 2)-, (4, 3)-, (5, 4)-, and (6, 8)-talisman squares.
-talisman square. The above illustrations show (4, 2)-, (4, 3)-, (5, 4)-, and (6, 8)-talisman squares.
See also Antimagic Square, Heterosquare, Magic Square, Talisman Hexagon
References
Madachy, J. S. Madachy's Mathematical Recreations. New York: Dover, pp. 110-113, 1979.
![]() Weisstein, E. W. ``Magic Squares.'' Mathematica notebook MagicSquares.m.
Weisstein, E. W. ``Magic Squares.'' Mathematica notebook MagicSquares.m.