Let  denote an
denote an 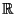 -algebra, so that
-algebra, so that  is a Vector Space over
is a Vector Space over 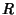 and
and
where 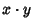 is vector multiplication which is assumed to be Bilinear. Now define
is vector multiplication which is assumed to be Bilinear. Now define
where 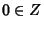 .
.  is said to be tame if
is said to be tame if 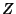 is a finite union of Subspaces of
is a finite union of Subspaces of  . A 2-D 0-Associative
algebra is tame, but a 4-D 4-Associative algebra and a 3-D 1-Associative algebra need not be tame. It is
conjectured that a 3-D 2-Associative algebra is tame, and proven that a 3-D 3-Associative algebra is tame
if it possesses a multiplicative Identity Element.
. A 2-D 0-Associative
algebra is tame, but a 4-D 4-Associative algebra and a 3-D 1-Associative algebra need not be tame. It is
conjectured that a 3-D 2-Associative algebra is tame, and proven that a 3-D 3-Associative algebra is tame
if it possesses a multiplicative Identity Element.
References
Finch, S. ``Zero Structures in Real Algebras.''
http://www.mathsoft.com/asolve/zerodiv/zerodiv.html.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() denote an
denote an ![]() -algebra, so that
-algebra, so that ![]() is a Vector Space over
is a Vector Space over ![]() and
and