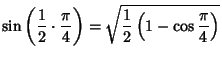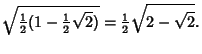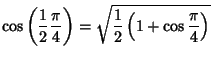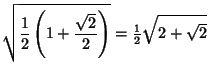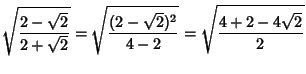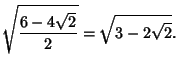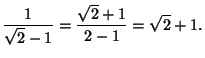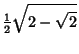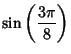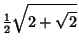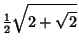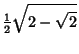Now, checking to see if the Square Root can be simplified gives
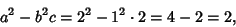 |
(2) |
which is not a Perfect Square, so the above expression cannot be simplified. Similarly,
But
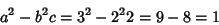 |
(5) |
is a Perfect Square, so we can find
 |
(6) |
Rewrite the above as
Summarizing,
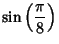 |
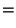 |
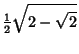 |
(9) |
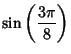 |
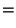 |
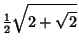 |
(10) |
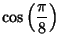 |
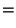 |
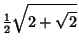 |
(11) |
 |
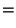 |
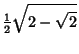 |
(12) |
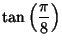 |
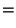 |
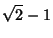 |
(13) |
 |
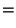 |
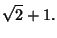 |
(14) |
See also Octagon
© 1996-9 Eric W. Weisstein
1999-05-26