|
|
|
Rather surprisingly, trigonometric functions of ![]() for
for ![]() an integer can be expressed in terms of sums, products, and
finite root extractions because 17 is a Fermat Prime. This makes the Heptadecagon a
Constructible, as first proved by Gauß.
an integer can be expressed in terms of sums, products, and
finite root extractions because 17 is a Fermat Prime. This makes the Heptadecagon a
Constructible, as first proved by Gauß. ![]() Although Gauss did not actually
explicitly provide a construction, he did derive the trigonometric formulas below using a series of intermediate variables
from which the final expressions were then built up.
Although Gauss did not actually
explicitly provide a construction, he did derive the trigonometric formulas below using a series of intermediate variables
from which the final expressions were then built up.
Let
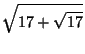 |
|||
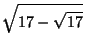 |
|||
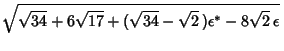 |
|||
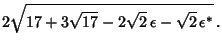 |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
There are some interesting analytic formulas involving the trigonometric functions of ![]() . Define
. Define
![\begin{eqnarray*}
P(x)&\equiv&(x-1)(x-2)(x^2+1)\\
g_1(x)&\equiv& {2+\sqrt{P(x...
...r 4}}[g_i(x)-1]\\
a&\equiv& {\textstyle{1\over 4}}\tan^{-1} 4,
\end{eqnarray*}](t_2127.gif)
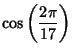 |
|||
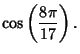 |
See also Constructible Polygon, Fermat Prime, Heptadecagon
References
Casey, J. Plane Trigonometry. Dublin: Hodges, Figgis, & Co., p. 220, 1888.
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, pp. 192-194 and 229-230, 1996.
Dörrie, H. ``The Regular Heptadecagon.'' §37 in
100 Great Problems of Elementary Mathematics: Their History and Solutions. New York: Dover, pp. 177-184, 1965.
Ore, Ø. Number Theory and Its History. New York: Dover, 1988.
Smith, D. E. A Source Book in Mathematics. New York: Dover, p. 348, 1994.
|
|
|
© 1996-9 Eric W. Weisstein