|
|
|
The Space Curve giving the intersection of the Cylinder
| (1) |
| (2) |
| (3) | |||
| (4) | |||
| (5) |
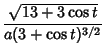 |
(6) | ||
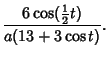 |
(7) |
See also Cylinder, Sphere, Steinmetz Solid
References
Gray, A. ``Viviani's Curve.'' §7.6 in Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, pp. 140-142, 1993.
von Seggern, D. CRC Standard Curves and Surfaces. Boca Raton, FL: CRC Press, p. 270, 1993.