The Tensor
where 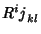 is the Riemann Tensor and
is the Riemann Tensor and 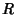 is the Curvature Scalar. The Weyl tensor is defined so that
every Contraction between indices gives 0. In particular,
is the Curvature Scalar. The Weyl tensor is defined so that
every Contraction between indices gives 0. In particular,
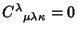 . The number of independent components for a Weyl tensor in
. The number of independent components for a Weyl tensor in 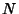 -D is given by
-D is given by
See also Curvature Scalar, Riemann Tensor
References
Weinberg, S. Gravitation and Cosmology: Principles and Applications of the General Theory of Relativity.
New York: Wiley, p. 146, 1972.
© 1996-9 Eric W. Weisstein
1999-05-26