Some interesting properties (as well as a few arcane ones not reiterated here) of the number 239 are discussed in
Beeler et al. (1972, Item 63). 239 appears in Machin's Formula
which is related to the fact that
which is why 239/169 is the 7th Convergent of 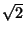 . Another pair of Inverse Tangent
Formulas involving 239 is
. Another pair of Inverse Tangent
Formulas involving 239 is
239 needs 4 Squares (the maximum) to express it, 9 Cubes (the maximum,
shared only with 23) to express it, and 19 fourth Powers (the maximum) to express it (see Waring's
Problem). However, 239 doesn't need the maximum number of fifth Powers (Beeler et al. 1972, Item 63).
References
Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT
Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972.
© 1996-9 Eric W. Weisstein
1999-05-25