|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Let ![]() be the number of ways in which the Factorial
be the number of ways in which the Factorial ![]() can be decomposed into
can be decomposed into ![]() Factors of
the form
Factors of
the form ![]() arranged in nondecreasing order. Also define
arranged in nondecreasing order. Also define
| (1) |
| (2) |
| (3) |
| (4) |
| (5) |
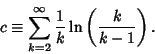 |
(6) |
References
Alladi, K. and Grinstead, C. ``On the Decomposition of
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/aldgrns/aldgrns.html
Guy, R. K. ``Factorial
![]() into Prime Powers.'' J. Number Th. 9,
452-458, 1977.
into Prime Powers.'' J. Number Th. 9,
452-458, 1977.
![]() as the Product of
as the Product of ![]() Large Factors.'' §B22 in
Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, p. 79, 1994.
Large Factors.'' §B22 in
Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, p. 79, 1994.
|
|
|
© 1996-9 Eric W. Weisstein