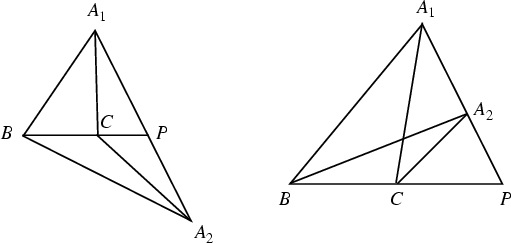
The ``Area principle'' states that
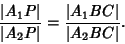 |
(1) |
This can also be written in the form
![\begin{displaymath}
\left[{A_1P\over A_2P}\right]=\left[{A_1BC\over A_2BC}\right],
\end{displaymath}](a_1557.gif) |
(2) |
where
![\begin{displaymath}
\left[{AB\over CD}\right]
\end{displaymath}](a_1558.gif) |
(3) |
is the ratio of the lengths ![$[A, B]$](a_1559.gif) and
and ![$[C, D]$](a_1560.gif) for
for 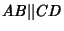 with a Plus or Minus Sign
depending on if these segments have the same or opposite directions, and
with a Plus or Minus Sign
depending on if these segments have the same or opposite directions, and
![\begin{displaymath}
\left[{ABC\over DEFG}\right]
\end{displaymath}](a_1562.gif) |
(4) |
is the Ratio of signed Areas of the Triangles. Grünbaum and Shepard show that
Ceva's Theorem, Hoehn's Theorem, and Menelaus' Theorem are the consequences of this result.
See also Ceva's Theorem, Hoehn's Theorem, Menelaus' Theorem, Self-Transversality Theorem
References
Grünbaum, B. and Shepard, G. C. ``Ceva, Menelaus, and the Area Principle.'' Math. Mag. 68, 254-268,
1995.
© 1996-9 Eric W. Weisstein
1999-05-25