|
|
|
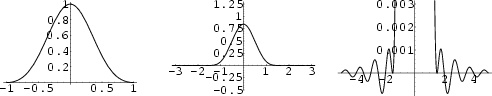
An Apodization Function given by
| (1) |
| (2) |
| (3) | |||
| (4) | |||
| (5) |
See also Apodization Function
References
Blackman, R. B. and Tukey, J. W. ``Particular Pairs of Windows.'' In The Measurement of Power Spectra, From the
Point of View of Communications Engineering. New York: Dover, pp. 98-99, 1959.