|
|
|
The Cantor set (![]() ) is given by taking the interval [0,1] (set
) is given by taking the interval [0,1] (set ![]() ), removing the middle third (
), removing the middle third (![]() ), removing
the middle third of each of the two remaining pieces (
), removing
the middle third of each of the two remaining pieces (![]() ), and continuing this procedure ad infinitum. It is therefore
the set of points in the Interval [0,1] whose ternary expansions do not contain 1,
illustrated below.
), and continuing this procedure ad infinitum. It is therefore
the set of points in the Interval [0,1] whose ternary expansions do not contain 1,
illustrated below.
This produces the Set of Real Numbers ![]() such that
such that
| (1) |
| (2) |
| (3) |
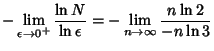 |
|||
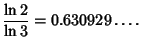 |
(4) |
A general Cantor set is a Closed Set consisting entirely of Boundary Points. Such sets are Uncountable and may have 0 or Positive Lebesgue Measure. The Cantor set is the only totally disconnected, perfect, Compact Metric Space up to a Homeomorphism (Willard 1970).
See also Alexander's Horned Sphere, Antoine's Necklace, Cantor Function
References
Boas, R. P. Jr. A Primer of Real Functions. Washington, DC: Amer. Math. Soc., 1996.
Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press,
pp. 15-20, 1991.
Willard, S. §30.4 in General Topology. Reading, MA: Addison-Wesley, 1970.
|
|
|
© 1996-9 Eric W. Weisstein