|
|
|
Consider a point light source located at a point ![]() . The Catacaustic of a
unit Circle for the light at
. The Catacaustic of a
unit Circle for the light at ![]() is the Nephroid
is the Nephroid
| (1) | |||
| (2) |
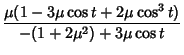 |
(3) | ||
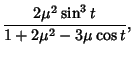 |
(4) |
| (5) | |||
| (6) |
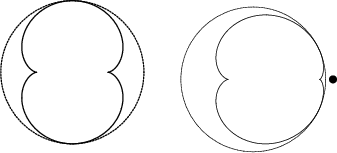
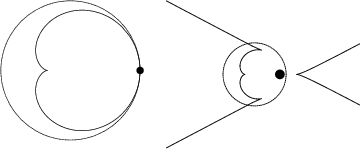
The Catacaustic for Parallel rays crossing a Circle is a Cardioid.
See also Catacaustic, Caustic