|
|
|
A Sequence of numbers ![]() is complete if every Positive Integer
is complete if every Positive Integer ![]() is the sum of some
subsequence of
is the sum of some
subsequence of ![]() , i.e., there exist
, i.e., there exist ![]() or 1 such that
or 1 such that
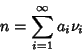
See also Bertrand's Postulate, Brown's Criterion, Fibonacci Dual Theorem, Greedy Algorithm, Weakly Complete Sequence, Zeckendorf's Theorem
References
Brown, J. L. Jr. ``Unique Representations of Integers as Sums of Distinct Lucas Numbers.'' Fib. Quart. 7, 243-252, 1969.
Hoggatt, V. E. Jr.; Cox, N.; and Bicknell, M. ``A Primer for Fibonacci Numbers. XII.'' Fib. Quart. 11, 317-331, 1973.
Honsberger, R. Mathematical Gems III. Washington, DC: Math. Assoc. Amer., 1985.