|
|
|
Sums with unity Numerator and Factorials in the Denominator which can be expressed analytically include
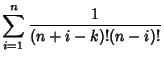 |
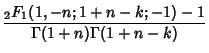 |
(1) | |
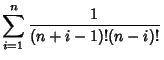 |
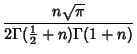 |
(2) | |
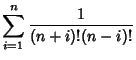 |
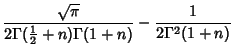 |
(3) | |
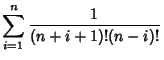 |
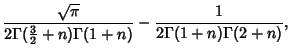 |
(4) |
Sums with ![]() in the Numerator having analytic solutions include
in the Numerator having analytic solutions include
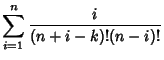 |
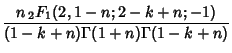 |
(5) | |
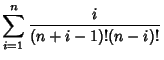 |
![$\displaystyle {1\over 2\Gamma(n)} \left[{{\sqrt{\pi}\over 2\Gamma({\textstyle{1\over 2}}+n)}+{n\over\Gamma(1+n)}}\right]$](f_210.gif) |
(6) | |
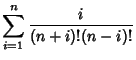 |
(7) | ||
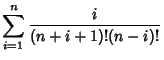 |
![$\displaystyle {1\over 2\Gamma(1+n)}\left[{{1\over\Gamma(2+n)}-{(n^2+3n+2)\sqrt{\pi}\over 2\Gamma({\textstyle{3\over 2}}+n)}\,}\right].$](f_214.gif) |
(8) |
|
|
|
|
|
|
|
|
(9) |
Sums of factorial Powers include
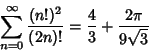 |
(10) |
![\begin{displaymath}
\sum_{n=0}^\infty {(n!)^3\over(3n)!}=\int_0^1 [P(t)+Q(t)\cos^{-1} R(t)]\,dt,
\end{displaymath}](f_220.gif) |
(11) |
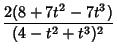 |
(12) | ||
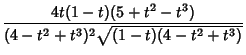 |
(13) | ||
| (14) |
References
Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972.
|
|
|
© 1996-9 Eric W. Weisstein