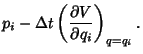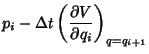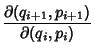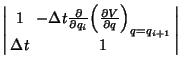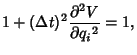Consider a 1-D Hamiltonian Map of the form
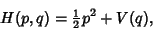 |
(1) |
which satisfies Hamilton's Equations
Now, write
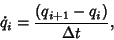 |
(4) |
where
Then the equations of motion become
Note that equations (7) and (8) are not
Area-Preserving, since
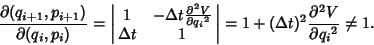 |
(9) |
However, if we take instead of (7) and (8),
which is Area-Preserving.
© 1996-9 Eric W. Weisstein
1999-05-25