|
|
|
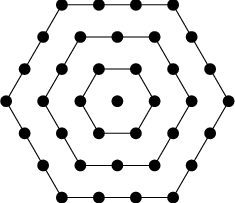
The Centered Hexagonal Number given by
The first Triangular hex numbers are 1 and 91, and the first few Square ones are 1,
169, 32761, 6355441, ... (Sloane's A006051). Square hex numbers are obtained by solving the
Diophantine Equation
See also Magic Hexagon, Centered Square Number, Star Number, Talisman Hexagon
References
Conway, J. H. and Guy, R. K. The Book of Numbers. New York: Springer-Verlag, p. 41, 1996.
Gardner, M. ``Hexes and Stars.'' Ch. 2 in Time Travel and Other Mathematical Bewilderments.
New York: W. H. Freeman, 1988.
Hindin, H. ``Stars, Hexes, Triangular Numbers, and Pythagorean Triples.'' J. Recr. Math. 16, 191-193, 1983-1984.
Sloane, N. J. A. Sequences
A003215/M4362
and A006051/M5409
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.