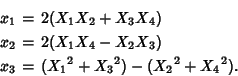|
|
|
The first example discovered of a Map from a higher-dimensional Sphere to a lower-dimensional Sphere
which is not null-Homotopic. Its discovery was a shock to the mathematical community, since it was
believed at the time that all such maps were null-Homotopic, by analogy with Homology
Groups. The Hopf map takes points (![]() ,
, ![]() ,
, ![]() ,
, ![]() ) on a 3-sphere to points on a 2-sphere
(
) on a 3-sphere to points on a 2-sphere
(![]() ,
, ![]() ,
, ![]() )
)