|
|
|
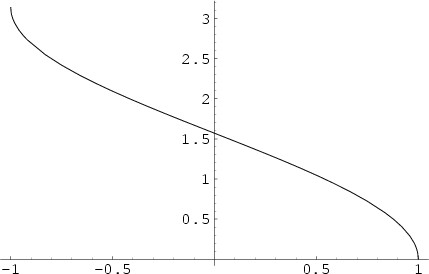
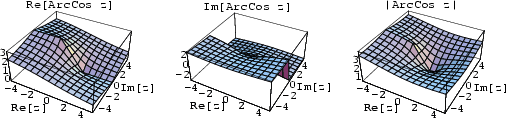
The function ![]() , also denoted arccos(
, also denoted arccos(![]() ), where
), where ![]() is the Cosine and the superscript
is the Cosine and the superscript ![]() denotes an
Inverse Function, not the multiplicative inverse. The Maclaurin Series for the inverse cosine range
denotes an
Inverse Function, not the multiplicative inverse. The Maclaurin Series for the inverse cosine range
![]() is
is
| (1) |
| (2) |
| (3) |
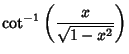 |
(4) | ||
| (5) | |||
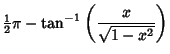 |
(6) |
 |
(7) | ||
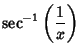 |
(8) | ||
| (9) | |||
 |
(10) |
See also Cosine, Inverse Secant
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Inverse Circular Functions.'' §4.4 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 79-83, 1972.
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, pp. 142-143, 1987.
|
|
|
© 1996-9 Eric W. Weisstein