|
|
|
There are several functions called ``Lommel functions.'' One type of Lommel function is the solution to the Lommel
Differential Equation with a Plus Sign,
| (1) |
| (2) |
| (3) |
Lommel functions of two variables are related to the Bessel Function of the First Kind and arise in the theory of
diffraction ![]() and, in particular, Mie scattering
and, in particular, Mie scattering ![]() (Watson 1966, p. 537),
(Watson 1966, p. 537),
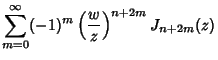 |
(4) | ||
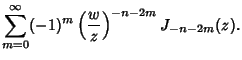 |
(5) |
See also Lommel Differential Equation, Lommel Polynomial
References
Chandrasekhar, S. Radiative Transfer. New York: Dover, p. 369, 1960.
Watson, G. N. A Treatise on the Theory of Bessel Functions, 2nd ed. Cambridge, England: Cambridge University Press, 1966.