|
|
|
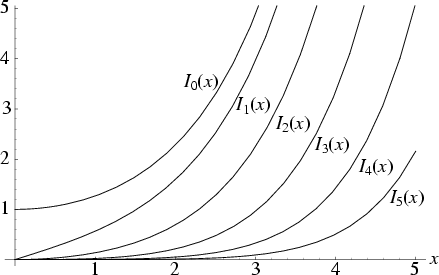
A function ![]() which is one of the solutions to the Modified Bessel Differential Equation and is closely
related to the Bessel Function of the First Kind
which is one of the solutions to the Modified Bessel Differential Equation and is closely
related to the Bessel Function of the First Kind ![]() . The above plot shows
. The above plot shows ![]() for
for ![]() , 2, ..., 5. In
terms of
, 2, ..., 5. In
terms of
![]() ,
,
| (1) |
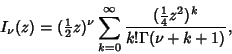 |
(2) |
| (3) |
| (4) |
A derivative identity for expressing higher order modified Bessel functions in terms of ![]() is
is
| (5) |
See also Bessel Function of the First Kind, Modified Bessel Function of the First Kind, Weber's Formula
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Modified Bessel Functions
Arfken, G. ``Modified Bessel Functions,
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/cntfrc/cntfrc.html
Press, W. H.; Flannery, B. P.; Teukolsky, S. A.; and Vetterling, W. T.
``Bessel Functions of Fractional Order, Airy Functions, Spherical Bessel Functions.'' §6.7 in
Numerical Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. Cambridge, England:
Cambridge University Press, pp. 234-245, 1992.
Spanier, J. and Oldham, K. B. ``The Hyperbolic Bessel Functions
![]() and
and ![]() .''
§9.6 in Handbook of Mathematical Functions with Formulas,
Graphs, and Mathematical Tables, 9th printing. New York: Dover,
pp. 374-377, 1972.
.''
§9.6 in Handbook of Mathematical Functions with Formulas,
Graphs, and Mathematical Tables, 9th printing. New York: Dover,
pp. 374-377, 1972.
![]() and
and ![]() .'' §11.5 in
Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 610-616, 1985.
.'' §11.5 in
Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 610-616, 1985.
![]() and
and ![]() '' and
``The General Hyperbolic Bessel Function
'' and
``The General Hyperbolic Bessel Function ![]() .''
Chs. 49-50 in An Atlas of Functions. Washington, DC: Hemisphere,
pp. 479-487 and 489-497, 1987.
.''
Chs. 49-50 in An Atlas of Functions. Washington, DC: Hemisphere,
pp. 479-487 and 489-497, 1987.
|
|
|
© 1996-9 Eric W. Weisstein