|
|
|
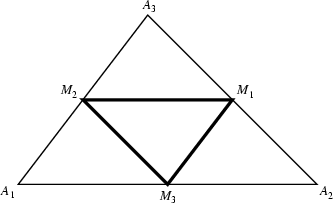
The Triangle
![]() formed by joining the Midpoints of the sides of a
Triangle
formed by joining the Midpoints of the sides of a
Triangle
![]() . The medial triangle is sometimes also called the Auxiliary Triangle
(Dixon 1991). The medial triangle has Trilinear Coordinates
. The medial triangle is sometimes also called the Auxiliary Triangle
(Dixon 1991). The medial triangle has Trilinear Coordinates
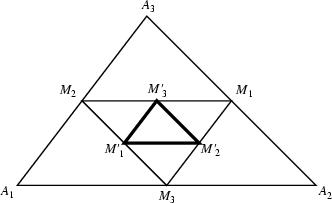
See also Anticomplementary Triangle
References
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 18-20,
1967.
Dixon, R. Mathographics. New York: Dover, p. 56, 1991.