Mid-Arc Points

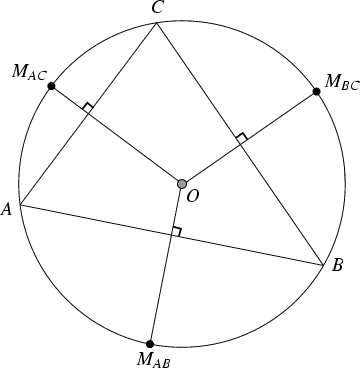
The mid-arc points $M_{AB}$, $M_{AC}$, and $M_{BC}$ of a Triangle $\Delta ABC$ are the points on the Circumcircle of the triangle which lie half-way along each of the three Arcs determined by the vertices (Johnson 1929). These points arise in the definition of the Fuhrmann Circle and Fuhrmann Triangle, and lie on the extensions of the Perpendicular Bisectors of the triangle sides drawn from the Circumcenter $O$.

Kimberling (1988, 1994) and Kimberling and Veldkamp (1987) define the mid-arc points as the Points which have Triangle Center Functions

$$\alpha_1 = [\cos({\textstyle{1\over 2}}B)+\cos({\textstyle{1\over 2}}C)]\sec({\textstyle{1\over 2}}A)$$

$$\alpha_2 = [\cos({\textstyle{1\over 2}}B)+\cos({\textstyle{1\over 2}}C)]\csc({\textstyle{1\over 2}}A).$$

See also Fuhrmann Circle, Fuhrmann Triangle

References

Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA: Houghton Mifflin, pp. 228-229, 1929.

Kimberling, C. ``Problem 804.'' Nieuw Archief voor Wiskunde 6, 170, 1988.

Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994.

Kimberling, C. and Veldkamp, G. R. ``Problem 1160 and Solution.'' Crux Math. 13, 298-299, 1987.