When 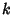 and
and 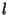 satisfy a Modular Equation, a relationship of the form
satisfy a Modular Equation, a relationship of the form
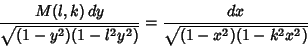 |
(1) |
exists, and 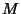 is called the multiplier. The multiplier of degree
is called the multiplier. The multiplier of degree 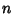 can be given by
can be given by
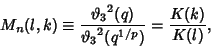 |
(2) |
where 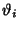 is a Theta Function and
is a Theta Function and 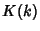 is a complete Elliptic Integral of the First Kind.
is a complete Elliptic Integral of the First Kind.
The first few multipliers in terms of 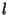 and
and 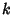 are
are
In terms of the 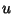 and
and 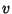 defined for Modular Equations,
defined for Modular Equations,
© 1996-9 Eric W. Weisstein
1999-05-26
![]() and
and ![]() satisfy a Modular Equation, a relationship of the form
satisfy a Modular Equation, a relationship of the form
![]() and
and ![]() are
are
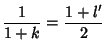

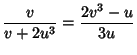
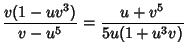
![$\displaystyle {v(1-uv)[1-uv+(uv)^2]\over v-u^7}$](m_1485.gif)
![$\displaystyle {v^7-u\over 7u(1-uv)[1-uv+(uv)^2]}.$](m_1486.gif)