|
|
|
A Finite Difference identity giving an interpolated value between tabulated points ![]() in terms of the first value
in terms of the first value
![]() and the Powers of the Forward Difference
and the Powers of the Forward Difference ![]() . For
. For ![]() , the formula states
, the formula states
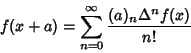
The Derivative of Newton's forward difference formula gives Markoff's Formulas.
See also Finite Difference, Markoff's Formulas, Newton's Backward Difference Formula, Newton's Divided Difference Interpolation Formula
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 880, 1972.
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 432, 1987.