|
|
|
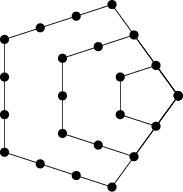
A Polygonal Number of the form ![]() . The first few are 1, 5, 12, 22, 35, 51, 70, ... (Sloane's A000326).
The Generating Function for the pentagonal numbers is
. The first few are 1, 5, 12, 22, 35, 51, 70, ... (Sloane's A000326).
The Generating Function for the pentagonal numbers is
The so-called generalized pentagonal numbers are given by ![]() with
with ![]() ,
, ![]() ,
, ![]() , ..., the first
few of which are 0, 1, 2, 5, 7, 12, 15, 22, 26, 35, ... (Sloane's A001318).
, ..., the first
few of which are 0, 1, 2, 5, 7, 12, 15, 22, 26, 35, ... (Sloane's A001318).
See also Euler's Pentagonal Number Theorem, Partition Function P, Polygonal Number, Triangular Number
References
Guy, R. K. ``Sums of Squares.'' §C20 in
Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, pp. 136-138, 1994.
Pappas, T. ``Triangular, Square & Pentagonal Numbers.'' The Joy of Mathematics.
San Carlos, CA: Wide World Publ./Tetra, p. 214, 1989.
Sloane, N. J. A. Sequences
A000326/M3818
and A001318/M1336
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.