|
|
|
Let ![]() be a Positive Integer and
be a Positive Integer and ![]() the number of (not necessarily distinct) Prime Factors of
the number of (not necessarily distinct) Prime Factors of ![]() (with
(with ![]() ). Let
). Let ![]() be the number of Positive Integers
be the number of Positive Integers ![]() with an Odd number of
Prime factors, and
with an Odd number of
Prime factors, and ![]() the number of Positive Integers
the number of Positive Integers ![]() with an Even number of
Prime factors. Pólya conjectured that
with an Even number of
Prime factors. Pólya conjectured that
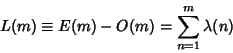
The conjecture was made in 1919, and disproven by Haselgrove (1958) using a method due to Ingham (1942). Lehman
(1960) found the first explicit counterexample,
![]() , and the smallest counterexample
, and the smallest counterexample
![]() was
found by Tanaka (1980). The first
was
found by Tanaka (1980). The first ![]() for which
for which ![]() are
are ![]() , 4, 6, 10, 16, 26, 40, 96, 586,
906150256, ... (Tanaka 1980, Sloane's A028488). It is unknown if
, 4, 6, 10, 16, 26, 40, 96, 586,
906150256, ... (Tanaka 1980, Sloane's A028488). It is unknown if ![]() changes sign infinitely often (Tanaka 1980).
changes sign infinitely often (Tanaka 1980).
See also Andrica's Conjecture, Liouville Function, Prime Factors
References
Haselgrove, C. B. ``A Disproof of a Conjecture of Pólya.'' Mathematika 5, 141-145, 1958.
Ingham, A. E. ``On Two Conjectures in the Theory of Numbers.'' Amer. J. Math. 64, 313-319, 1942.
Lehman, R. S. ``On Liouville's Function.'' Math. Comput. 14, 311-320, 1960.
Sloane, N. J. A. Sequence
A028488
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.
Tanaka, M. ``A Numerical Investigation on Cumulative Sum of the Liouville Function'' [sic]. Tokyo J. Math. 3, 187-189, 1980.