|
|
|
A Prime Constellation of four successive Primes with minimal distance
![]() . The quadruplet (2, 3, 5, 7)
has smaller minimal distance, but it is an exceptional special case. With the exception of (5, 7, 11, 13), a prime quadruple
must be of the form (
. The quadruplet (2, 3, 5, 7)
has smaller minimal distance, but it is an exceptional special case. With the exception of (5, 7, 11, 13), a prime quadruple
must be of the form (![]() ,
, ![]() ,
, ![]() ,
, ![]() ). The first few values of
). The first few values of ![]() which give prime quadruples are
which give prime quadruples are
![]() , 3, 6, 27, 49, 62, 69, 108, 115, ... (Sloane's A014561), and the first few values of
, 3, 6, 27, 49, 62, 69, 108, 115, ... (Sloane's A014561), and the first few values of ![]() are 5 (the exceptional case),
11, 101, 191, 821, 1481, 1871, 2081, 3251, 3461, .... The asymptotic Formula for the frequency of prime quadruples
is analogous to that for other Prime Constellations,
are 5 (the exceptional case),
11, 101, 191, 821, 1481, 1871, 2081, 3251, 3461, .... The asymptotic Formula for the frequency of prime quadruples
is analogous to that for other Prime Constellations,
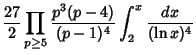 |
|||
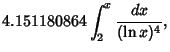 |
See also Prime Arithmetic Progression, Prime Constellation, Prime k-Tuples Conjecture, Sexy Primes, Twin Primes
References
Hardy, G. H. and Wright, E. M. An Introduction to the Theory of Numbers, 5th ed. New York: Oxford University Press, 1979.
Forbes, T. ``Prime
Rademacher, H. Lectures on Elementary Number Theory. New York: Blaisdell, 1964.
Riesel, H. Prime Numbers and Computer Methods for Factorization, 2nd ed. Boston, MA: Birkhäuser, pp. 61-62, 1994.
Roonguthai, W. ``Large Prime Quadruplets.''
http://www.mathsoft.com/asolve/constant/hrdyltl/roonguth.html.
Sloane, N. J. A. Sequence
A014561
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.
![]() -tuplets.''
http://www.ltkz.demon.co.uk/ktuplets.htm.
-tuplets.''
http://www.ltkz.demon.co.uk/ktuplets.htm.
|
|
|
© 1996-9 Eric W. Weisstein