|
|
|
A Spheroid which is ``pointy'' instead of ``squashed,'' i.e., one for which the polar radius ![]() is greater than
the equatorial radius
is greater than
the equatorial radius ![]() , so
, so ![]() . A prolate spheroid has Cartesian equations
. A prolate spheroid has Cartesian equations
| (1) |
| (2) |
| (3) |
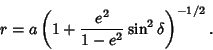 |
(4) |
| (5) | |||
| (6) |
See also Darwin-de Sitter Spheroid, Ellipsoid, Oblate Spheroid, Prolate Spheroidal Coordinates, Sphere, Spheroid
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 131, 1987.