A spheroid is an Ellipsoid
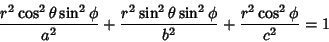 |
(1) |
with two Semimajor Axes equal. Orient the Ellipse so that the 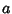 and
and 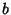 axes are
equal, then
axes are
equal, then
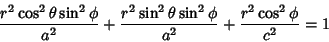 |
(2) |
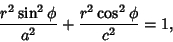 |
(3) |
where 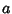 is the equatorial Radius and
is the equatorial Radius and 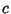 is the polar Radius. Here
is the polar Radius. Here 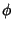 is the colatitude, so take
is the colatitude, so take
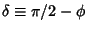 to express in terms of latitude.
to express in terms of latitude.
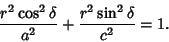 |
(4) |
Rewriting
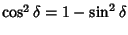 gives
gives
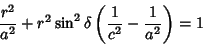 |
(5) |
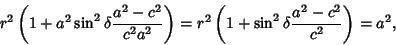 |
(6) |
so
 |
(7) |
If 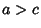 , the spheroid is Oblate. If
, the spheroid is Oblate. If 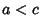 , the spheroid is Prolate. If
, the spheroid is Prolate. If 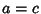 , the spheroid degenerates to a Sphere.
, the spheroid degenerates to a Sphere.
See also Darwin-de Sitter Spheroid, Ellipsoid, Oblate Spheroid, Prolate Spheroid
© 1996-9 Eric W. Weisstein
1999-05-26
