|
|
|
A curve named and studied by Newton ![]() in 1701 and contained in his classification of Cubic Curves. It had been studied earlier by L'Hospital
in 1701 and contained in his classification of Cubic Curves. It had been studied earlier by L'Hospital ![]() and Huygens
and Huygens ![]() in 1692 (MacTutor Archive).
in 1692 (MacTutor Archive).
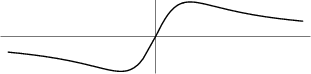
The curve is given by the Cartesian equation
| (1) |
| (2) | |||
| (3) |
The curve has a Maximum at ![]() and a Minimum at
and a Minimum at ![]() , where
, where
| (4) |
| (5) |
![$\displaystyle {2abx(x^2-3a^2)\over(x^2+a^2)^3\left[{1+{(a^3b-abx^2)^2\over(x^2+a^2)^4}}\right]^{3/2}}$](s1_786.gif) |
(6) | ||
![$\displaystyle -{4\sqrt{2}\,ab[2\cos(2t)-1]\cot t\csc^2 t\over \{b^2[1+\cos(4t)]+2a^2\csc^4 t\}^{3/2}}.$](s1_787.gif) |
(7) |
References
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 111-112, 1972.
MacTutor History of Mathematics Archive. ``Serpentine.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Serpentine.html.