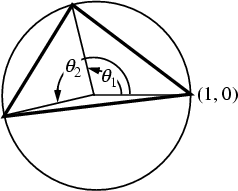
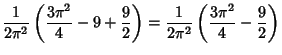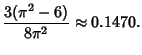Select three points at random on a unit Circle. Find the distribution of possible areas. The first point can be
assigned coordinates (1, 0) without loss of generality. Call the central angles from the first point to the second and
third 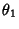 and
and 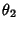 . The range of
. The range of 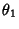 can be restricted to [0,
can be restricted to [0, 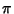 ] because of symmetry, but
] because of symmetry, but
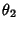 can range from
can range from 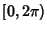 . Then
. Then
![\begin{displaymath}
A(\theta_1,\theta_2) =2\left\vert{\sin({\textstyle{1\over 2}...
...2)\sin[{\textstyle{1\over 2}}(\theta_1-\theta_2)]}\right\vert,
\end{displaymath}](t_1374.gif) |
(1) |
so
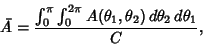 |
(2) |
where
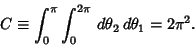 |
(3) |
Therefore,
But
Write (4) as
![\begin{displaymath}
\bar A={1\over\pi^2}\left[{\int_0^\pi \sin({\textstyle{1\ove...
...pi
\sin({\textstyle{1\over 2}}\theta_1)I_2\,d\theta_1}\right],
\end{displaymath}](t_1389.gif) |
(6) |
then
![\begin{displaymath}
I_1 \equiv \int_{\theta_1}^{2\pi}
\sin({\textstyle{1\over 2}...
..._2)\sin[{\textstyle{1\over 2}}(\theta_2-\theta_1)]\,d\theta_2,
\end{displaymath}](t_1390.gif) |
(7) |
and
![\begin{displaymath}
I_2 \equiv
\int_0^{\theta_1}\sin({\textstyle{1\over 2}}\theta_2)\sin[{\textstyle{1\over 2}}(\theta_1-\theta_2)]\,d\theta_2.
\end{displaymath}](t_1391.gif) |
(8) |
From (6),
so
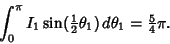 |
(10) |
Also,
so
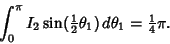 |
(12) |
Combining (10) and (12) gives
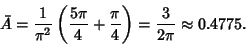 |
(13) |
The Variance is
See also Point-Point Distance--1-D, Tetrahedron Inscribing
© 1996-9 Eric W. Weisstein
1999-05-26
![]() and
and ![]() . The range of
. The range of ![]() can be restricted to [0,
can be restricted to [0, ![]() ] because of symmetry, but
] because of symmetry, but
![]() can range from
can range from ![]() . Then
. Then
![$\displaystyle {2\over 2\pi^2} \int_0^\pi\int_0^{2\pi}
\left\vert{\sin({\textsty...
...sin[{\textstyle{1\over 2}}(\theta_1-\theta_2)]}\right\vert d\theta_2\,d\theta_1$](t_1378.gif)
![$\displaystyle {1\over \pi^2} \int_0^\pi \sin({\textstyle{1\over 2}}\theta_1) \l...
...extstyle{1\over 2}}(\theta_2-\theta_1)]}\right\vert\,d\theta_2}\right]d\theta_1$](t_1379.gif)
![$\displaystyle {1\over \pi^2} {\int_0^\pi\int_0^{2\pi}\atop \scriptstyle\theta_2...
...}\theta_2)\sin[{\textstyle{1\over 2}}(\theta_1-\theta_2)]\,d\theta_2\,d\theta_1$](t_1380.gif)
![$\displaystyle + {1\over \pi^2} {\int_0^\pi\int_0^{2\pi}\atop\scriptstyle\theta_...
...}\theta_2)\sin[{\textstyle{1\over 2}}(\theta_1-\theta_2)]\,d\theta_2\,d\theta_1$](t_1381.gif)
![$\displaystyle {1\over \pi^2} \int_0^\pi \sin({\textstyle{1\over 2}}\theta_1) \l...
..._2)
\sin[{\textstyle{1\over 2}}(\theta_2-\theta_1)]\,d\theta_2}\right]d\theta_1$](t_1382.gif)
![$\displaystyle +{1\over \pi^2} \int_0^\pi \sin({\textstyle{1\over 2}}\theta_1) \...
...2)
\sin[{\textstyle{1\over 2}}(\theta_2-\theta_1)]\,d\theta_2}\right]d\theta_1.$](t_1383.gif)
![$\displaystyle {1\over 2\pi^2} \int_0^\pi \int_0^{2\pi} [A(\theta_1,\theta_2)-{\textstyle{3\over 2\pi}}]^2\,d\theta_2\,d\theta_1$](t_1407.gif)
![$\displaystyle {1\over 2\pi^2} \int_0^\pi \int_0^{2\pi}\left[{2\left\vert{\sin({...
...}(\theta_1-\theta_2)]}\right\vert-{3\over 2\pi}}\right]^2\,d\theta_2\,d\theta_1$](t_1408.gif)
![$\displaystyle {1\over 2\pi^2}\int_0^\pi\int_0^{2\pi}\left\{{4\sin^2({\textstyle...
...le{1\over 2}}\theta_2)\sin^2[{\textstyle{1\over 2}}(\theta_2-\theta_1)]}\right.$](t_1409.gif)
![$\displaystyle \left.{-{6\over\pi}\left\vert{\sin({\textstyle{1\over 2}}\theta_1...
...\theta_1-\theta_2)]}\right\vert +{9\over 4\pi^2}}\right\}\,d\theta_2\,d\theta_1$](t_1410.gif)
![$\displaystyle {1\over 2\pi^2}\left[{\int_0^\pi \pi(2+\theta_1)\sin^2({\textstyl...
...r \pi}\left({{5\pi\over 4}+{\pi\over 4}}\right)+{9\over 4\pi^2}(2\pi^2)}\right]$](t_1411.gif)