|
|
|
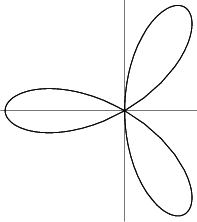
Lawrence (1972) defines a trifolium as a Folium with ![]() . However, the term ``the'' trifolium is
sometimes applied to the Folium with
. However, the term ``the'' trifolium is
sometimes applied to the Folium with ![]() , which is then the 3-petalled Rose with Cartesian equation
, which is then the 3-petalled Rose with Cartesian equation
See also Bifolium, Folium, Quadrifolium
References
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 152-153, 1972.
MacTutor History of Mathematics Archive. ``Trifolium.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Trifolium.html.