|
|
|
| (1) |
| (2) |
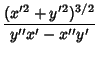 |
|||
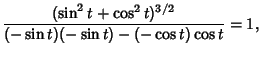 |
(3) |
| (4) |
| (5) | |||
| (6) |
| (7) | |||
| (8) |
See also Circle Involute
References
Gray, A. Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, p. 77, 1993.
Lauwerier, H. Fractals: Endlessly Repeated Geometric Figures. Princeton, NJ: Princeton University Press,
pp. 55-59, 1991.