|
|
|
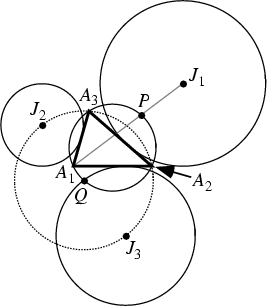
Given a Triangle
![]() , the points
, the points ![]() ,
, ![]() , and
, and ![]() lie on a line, where
lie on a line, where ![]() is the Incenter
and
is the Incenter
and ![]() is the Excenter corresponding to
is the Excenter corresponding to ![]() . Furthermore, the circle with
. Furthermore, the circle with ![]() as the diameter has
as the diameter has ![]() as its
center, where
as its
center, where ![]() is the intersection of
is the intersection of ![]() with the Circumcircle of
with the Circumcircle of ![]() and
and ![]() is the point opposite
is the point opposite ![]() on the Circumcircle. The circle with diameter
on the Circumcircle. The circle with diameter ![]() also passes through
also passes through ![]() and
and ![]() and has radius
and has radius
See also Excenter, Incenter-Excenter Circle, Orthocentric System
References
Johnson, R. A. Modern Geometry: An Elementary Treatise on the Geometry of the Triangle and the Circle. Boston, MA:
Houghton Mifflin, pp. 185-186, 1929.